non-abelian Yang monopole
Spinor BEC can quantum simulate a Yang monopole in 5D parameter space. How does the interaction affects the topological defects?
Definition
nLab described the Yang monopole in a mathematical way. In short, we could view Yang monopole as the generalization of the Dirac monopole from 3+1 dimensional spacetime to 5+1 dimensional spacetime.
Comparison to Dirac monopole
The Dirac monopole lives in 3D space and has a non-trivial first Chern number. The Yang monopoles lives in 5D space and has a non-trivial second Chern number with zero first Chern number. The Dirac monopole deals with U(1) gauge fields (scalar) whereas Yang monopole deals with non-abelian SU(2) gauge fields (matrix). Under U(1) gauge fields, the Berry phase is a scalar, if you adiabatically traverse a state along a closed loop, one could obtain an additional U(1) phase. Under SU(2) gauge fields, the Berry phase is a matrix, a spin up could become a spin down after the same process.
Synthetic dimension
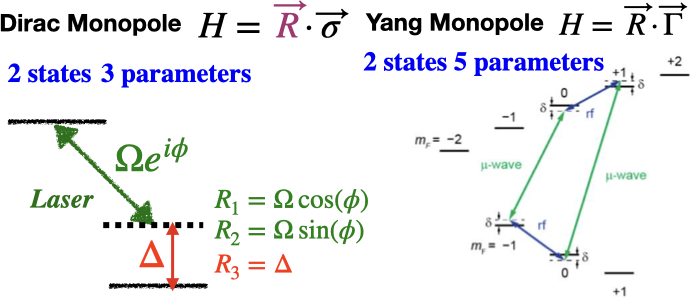
Even though a magnetic monopole (or Dirac monopole) does not exist in nature. One could still engineer a 3D synthetic magnetic field and simulate a Dirac monopole. A Yang Monopole lives in 5D. We have to use synthetic dimension. Here, one could use 5D parameter space. That is, 5 dimensions consist of laser intensities, phases, and detuning. We often use coupled two state model to describe a spin half on a Bloch sphere (equivalent to a Dirac monopole in the origin). One need a S4 Bloch sphere to enclose a Yang monopole. That is, one need a spin 3/2 or four states. Sugawa et. al., Science 360, 1429 (2018) simulated a Yang monopole by cyclically couple 4 internal states of a spinor BEC.
Effect of interaction
Atoms weakly interact, we would like to investigate how and if interaction changes the physical picture. When the interaction is weak, the non-interaction picture works well. But when the interaction is strong, we found that Yang monopole could split or high dimensional topological defects other than point defect could arise.